
IGNOU MST-002 Solved Assignment 2024 | PGDAST
₹351.00
Access via our Android App Only
Please read the following points before ordering :
Share with your Friends
Get the most comprehensive and accurate solution to the MST-002 assignment for the PGDAST program with our expert-written and verified MST-002 Solved Assignment 2024. This assignment covers all the questions with concepts of the MST-002 course and will help you score high grades in your examination. Our MST-002 Solved Assignment 2024 is a complete guide that includes step-by-step answers to all the questions and problems in the assignment, along with relevant examples and illustrations to make learning easier. This solution is written by experienced professionals who have a deep understanding of the subject and are well-versed in the latest course curriculum.
Our MST-002 Solved Assignment 2024 is designed to meet the requirements of IGNOU students and is available in a user-friendly format for easy access and use. The solution is available exclusively on our app and can be accessed from anywhere, anytime, on your Android device.
Don’t let the MST-002 assignment stress you out! Get the MST-002 Solved Assignment 2024 today and experience the ease of learning and improved grades.
Our MST-002 Solved Assignment 2024 is 100% original and free from plagiarism, ensuring that you receive a unique and authentic solution that meets the highest quality standards.
The solution is updated regularly to reflect any changes or updates in the course curriculum, ensuring that you receive the most current and accurate information.
With our MST-002 Solved Assignment 2024, you can save time and effort in completing your assignment, allowing you to focus on other important aspects of your studies.
Our team is available 24/7 to assist you with any questions or concerns you may have about the solution, ensuring that you receive the support you need to succeed.
The MST-002 Solved Assignment 2024 is reasonably priced and offers excellent value for money, helping you achieve your academic goals without breaking the bank.
By purchasing the MST-002 Solved Assignment 2024, you are investing in your academic success and taking the first step towards achieving your goals. Get your hands on the solution today and see the difference it can make in your studies!
IGNOU MST-002 Assignment Question Paper 2024
Get ahead in your IGNOU PGDAST Course studies with confidence by reviewing the MST-002 assignment questions before buying the expert-written solution from our app. Quick Search Section in our app makes it easy to double-check the year and session validity of the IGNOU assignment solutions, ensuring that you avoid any confusion. Our aim is to provide you with the most accurate and up-to-date IGNOU assignment solutions for PGDAST, and our expert-written assignments are unmatched by any other website. So, before making your purchase, take a moment to review the MST-002 assignment questions and ensure they match your needs. With our attention to detail and expertise, you can trust that you’re getting the right IGNOU assignment solution for your specific needs.
1. State whether the following statements are True or False and also give the reason in support of your answer.
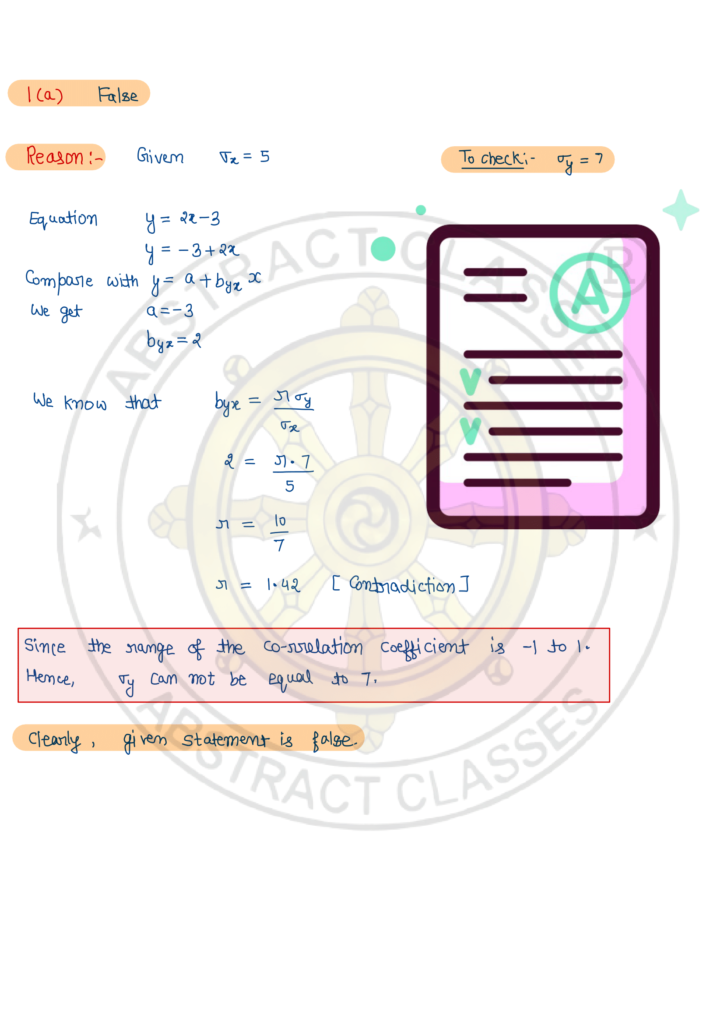
a) If standard deviation of \(x\) is 5 , standard deviation of \(y=2 x-3\) is 7 .
b) Mean deviation is least when calculated from the median.
c) The correlation coefficient between \(\mathrm{x}\) and \((a-\mathrm{x})\) is \(-1\).
d) The regression coefficients \(b_{y x}\) and \(b_{x y}\) of a data are \(1.2\) and \(0.8\), respectively.
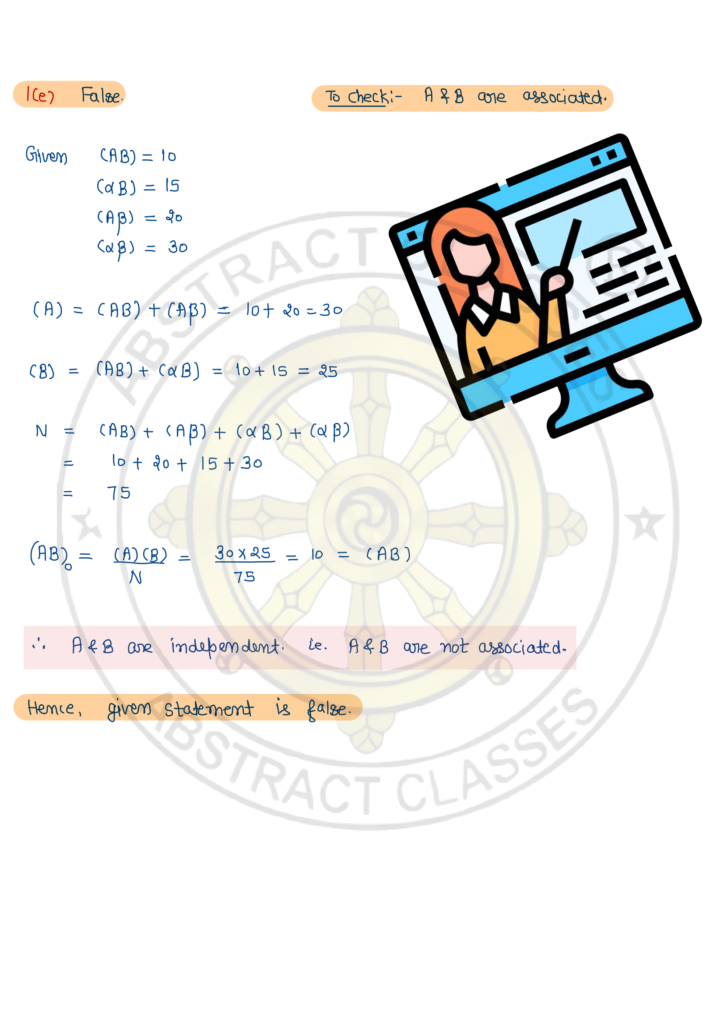
e) If \((\mathrm{AB})=10,(\alpha \mathrm{B})=15,(\mathrm{~A} \beta)=20\) and \((\alpha \beta)=30\) then \(\mathrm{A}\) and \(\mathrm{B}\) are associated.
2. a) Find the missing information from the following data:
\(\begin{array}{lcccl} & \text { Group I } & \text { Group II } & \text { Group III } & \text { Combined } \\ \text { Number } & 50 & ? & 90 & 200 \\ \text { Standard Deviation } & 6 & 7 & ? & 7.746 \\ \text { Mean } & 113 & ? & 115 & 116\end{array}\)
b) If AM and GM of two numbers are 30 and 18, respectively, find the numbers.
3. a) The frequency distribution of the marks obtained by the 25 students each of the two sections is given as follows:
\(\begin{array}{llllll}\text { Marks: } & 10-20 & 20-30 & 30-40 & 40-50 & 50-60 \\ \text { Section A: } & 2 & 5 & 10 & 5 & 3 \\ \text { Section B: } & 3 & 7 & 8 & 5 & 2\end{array}\)
Find which section is more consistent.
b) Mean and Standard deviation of 18 observations are found to be 7 and 4, respectively. On comparing the original data, it was found that an observation 12 was miscopied as 21 in the calculations. Calculate correct mean and standard deviation.
4. The equations of two regression lines are given as follows:
\[
\begin{aligned}
& 4 x-5 y+30=0 \\
& 20 x-9 y-107=0
\end{aligned}
\]
Calculate (i) regression coefficients, \(b_{y x}\) and \(b_{x y}\); (ii) correlation coefficient \(r(x, y)\); (iii) Mean of \(X\) and \(Y\); and (iv) the value of \(\sigma_{y}\) if \(\sigma_{x}=3\).
5. A researcher collects the following information for two variables \(\mathrm{x}\) and \(\mathrm{y}\) : \(\mathrm{n}=20, \mathrm{r}=0.5, \operatorname{mean}(\mathrm{x})=15\), mean \((\mathrm{y})=20, \sigma_{\mathrm{x}}=4\) and \(\sigma_{\mathrm{y}}=5\)
Later it was found that one pair of values \((\mathrm{x}, \mathrm{y})\) has been wrongly taken as \((16,30)\) whereas the correct values were \((26,35)\). Find the correct value of \(r(x, y)\). 6. a) If a, b, c, \(d\) are constants, then show that the coefficient of correlation between \(a x+b\) and \(c y+d\) is numerically equal to that between \(x\) and \(y\).
b) A statistician wanted to compare two methods A and B of teaching. He selected a random sample of 22 students. He grouped them into 11 pairs so that the students in pair have approximately equal scores on an intelligence test. In each pair one student was taught by method A and the other by method B and examined after the course. The marks obtained by both methods are given as:
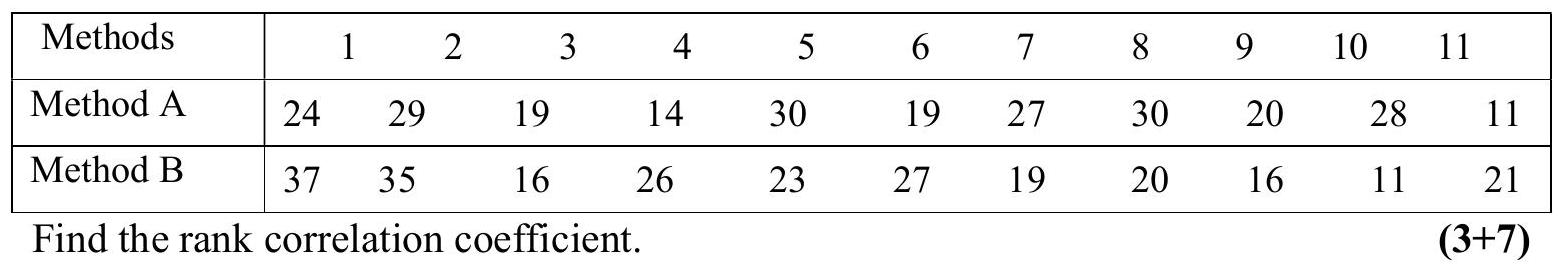
7. a) Fit an exponential curve of the form \(\mathrm{Y}=\mathrm{ab}^{\mathrm{X}}\) to the following data:
\(\begin{array}{lllllllll}\mathrm{X}: & 1 & 2 & 3 & 4 & 5 & 6 & 7 & 8\end{array}\)
\(\begin{array}{lllllllll}\mathrm{Y}: & 1.0 & 1.2 & 1.8 & 2.5 & 3.6 & 4.7 & 6.6 & 9.1\end{array}\)
b) Calculate the first, second and third quartile for the following data:
\(\begin{array}{lllllllll}\text { Class: } & \text { Below } 30 & 30-40 & 40-50 & 50-60 & 60-70 & 70-80 & 80 \text { and above }\end{array}\)
\(\begin{array}{llllllll}\text { Frequency: } & 69 & 167 & 207 & 65 & 58 & 27 & 10\end{array}\)
Also find the quartile deviation and coefficient of quartile deviation.
8. a) Board of Directors of Labour Union wishes to sample the opinion of its members before submitting a change in its contribution at a forthcoming annual meeting. Questionnaires are sent to a random sample of 200 members in three union locals. The results of the survey are as follows:
| Union Locals | ||||
| Reaction | A | B | C | Total |
| Favour Change | 35 | 45 | 20 | 100 |
| Against Change | 15 | 25 | 16 | 56 |
| No Response | 10 | 10 | 24 | 44 |
| Total | 60 | 80 | 60 | 200 |
Determine the amount of association between the Union locals and their reactions using coefficient of contingency and interpret the result.
b) 600 candidates were appeared in an examination. The boys outnumbered girls by \(15 \%\) of all candidates. Number of passed exceeded the number of failed candidates by 300 . Boys failing in the examination numbered 80. Determine the coefficient of association.
MST-002 Sample Solution 2024
Take a look at our sample solution of MST-002 Solved Assignment 2024 | PGDAST to get a feel for the top notch and accuracy of our IGNOU assignment solution. This sample solution is just one example of how we can help you with your IGNOU assignments, and we’re happy to present it as proof that we always produce high-quality work done by subject matter experts. Our assignments are solved by experts from multiple universities, unlike other sites that provide copied or stolen study materials. By doing so, we can guarantee that you will receive solutions that are both original and accurate, providing you with the knowledge you need to succeed academically. Don’t take our word for it; have a look at a sample of our MST-002 Solved Assignment 2024 | PGDAST now and see for yourself. You’ll have the advantage you need to excel in your IGNOU studies with our expert-written assignments.
1(a)
1(b)
True.
Mean deviation is least when calculated from the median.
The median is a measure of central tendency that divides the data into two equal halves, with half of the observations above the median and half below. The mean deviation from the median is calculated as the sum of the absolute deviations from the median divided by the number of observations.
Since the median separates the data into two equal halves, the sum of deviations from the median is zero, meaning that the mean deviation from the median is the smallest possible value for any measure of central tendency. This makes the median a robust statistic, meaning it is less sensitive to outliers in the data compared to other measures of central tendency like the mean.
Hence given statement is true.
1(c)
True.
The correlation coefficient between x and (a – x) is –1.
The correlation coefficient measures the strength and direction of the linear relationship between two variables. If x and (a – x) are perfectly negatively correlated, meaning that as x increases, (a – x) decreases and vice versa, then the correlation coefficient between the two variables will be equal to –1.
A correlation coefficient of –1 indicates a perfect negative linear relationship, where the variables are perfectly inversely proportional to each other. In this case, the variables x and (a – x) have a strong and perfect negative linear relationship, so the correlation coefficient between the two is –1.
Hence given statement is true.

Frequently Asked Questions (FAQs)
You can access the Complete Solution through our app, which can be downloaded using this link:
Simply click “Install” to download and install the app, and then follow the instructions to purchase the required assignment solution. Currently, the app is only available for Android devices. We are working on making the app available for iOS in the future, but it is not currently available for iOS devices.
Yes, It is Complete Solution, a comprehensive solution to the assignments for IGNOU. Valid from January 1, 2023 to December 31, 2023.
Yes, the Complete Solution is aligned with the IGNOU requirements and has been solved accordingly.
Yes, the Complete Solution is guaranteed to be error-free.The solutions are thoroughly researched and verified by subject matter experts to ensure their accuracy.
As of now, you have access to the Complete Solution for a period of 6 months after the date of purchase, which is sufficient to complete the assignment. However, we can extend the access period upon request. You can access the solution anytime through our app.
The app provides complete solutions for all assignment questions. If you still need help, you can contact the support team for assistance at Whatsapp +91-9958288900
No, access to the educational materials is limited to one device only, where you have first logged in. Logging in on multiple devices is not allowed and may result in the revocation of access to the educational materials.
Payments can be made through various secure online payment methods available in the app.Your payment information is protected with industry-standard security measures to ensure its confidentiality and safety. You will receive a receipt for your payment through email or within the app, depending on your preference.
The instructions for formatting your assignments are detailed in the Assignment Booklet, which includes details on paper size, margins, precision, and submission requirements. It is important to strictly follow these instructions to facilitate evaluation and avoid delays.
Terms and Conditions
- The educational materials provided in the app are the sole property of the app owner and are protected by copyright laws.
- Reproduction, distribution, or sale of the educational materials without prior written consent from the app owner is strictly prohibited and may result in legal consequences.
- Any attempt to modify, alter, or use the educational materials for commercial purposes is strictly prohibited.
- The app owner reserves the right to revoke access to the educational materials at any time without notice for any violation of these terms and conditions.
- The app owner is not responsible for any damages or losses resulting from the use of the educational materials.
- The app owner reserves the right to modify these terms and conditions at any time without notice.
- By accessing and using the app, you agree to abide by these terms and conditions.
- Access to the educational materials is limited to one device only. Logging in to the app on multiple devices is not allowed and may result in the revocation of access to the educational materials.
Our educational materials are solely available on our website and application only. Users and students can report the dealing or selling of the copied version of our educational materials by any third party at our email address (abstract4math@gmail.com) or mobile no. (+91-9958288900).
In return, such users/students can expect free our educational materials/assignments and other benefits as a bonafide gesture which will be completely dependent upon our discretion.
Related products
-
IGNOU Assignment Solution
IGNOU MEG-18 Solved Assignment 2022-2023 | MEG | American Poetry
₹101.00 Go to the App -
IGNOU Assignment Solution
IGNOU MEG-13 Solved Assignment 2022-2023 | MEG | WRITINGS FROM THE MARGINS
₹101.00 Go to the App -
IGNOU Assignment Solution
IGNOU MEG-08 Solved Assignment 2022-2023 | MEG | NEW LITERATURES IN ENGLISH
₹101.00 Go to the App -
IGNOU Assignment Solution
IGNOU MEG-05 Solved Assignment 2022-2023 | MEG | LITERARY CRITICISM & THEORY
₹101.00 Go to the App






